Put-Call Parity Explained: Why It Matters in Options Pricing



Put-call parity can help explain the relationship between call options, put options, and the underlying stock (or asset). If the formula doesn’t hold, there could be a potential pricing discrepancy. This concept is key for understanding fair value in options markets. It’s also closely linked to theBlack-Scholes model, which is used to price European-style options. In this guide, we’ll explain how put-call parity works in the context of options pricing.

The put-call parity formula 

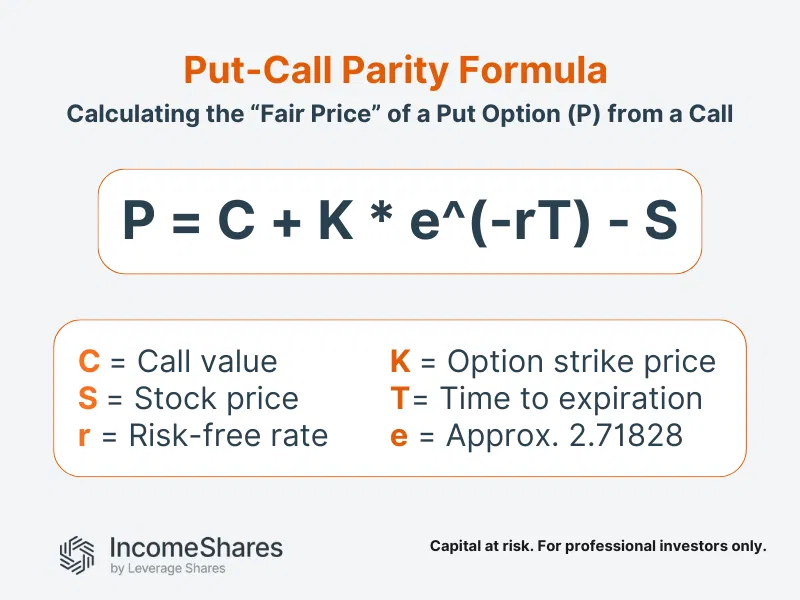
The formula is:

C + K × e^(-rT) = P + S

Where:

• C = Call option price

• P = Put option price

• S = Stock price (underlying asset)

• K = Strike price

• r = Risk-free interest rate

• T = Time to expiration

• e = Euler’s number (~2.71828)

An analyst might use the put-call parity formula to work out the “fair price” of a put option if they already know the price of a call option (or vice versa). If prices deviate from this relationship, there may be a pricing inefficiency in the market.



Put call parity example: working out the “fair price” of a put option 

Let’s assume a stock is trading at $100, and you know the following information:

• Call option price (C) = $10

• Strike price (K) = $95

• Risk-free rate (r) = 2%

• Time to expiration (T) = 1 year

Step 1: Rearrange the formula to solve for the price of the put option (P):

P = C – (S – K × e^(-rT))

Step 2: Plug in the values to get the result:

P = 10 – (100 – 95 × e^(-0.02)) = 10 – (100 – 95 × 0.9802) = 10 – (100 – 93.81)  = 10 – 6.19
P = 3.81

In this example, the fair price of the put option (P) should be $3.81, according to the put-call parity formula. If the actual market price differs, traders may explore potential opportunities, while considering the associated risks and market conditions.

How put-call parity links to the Black-Scholes Model 

The Black-Scholes model is a widely used formula for pricing European options. It assumes efficient markets and no early option exercise.

Put-call parity is built into Black-Scholes. If you plug a call price into Black-Scholes, you can rearrange the equation to get the put price (or vice versa). This helps maintain pricing consistency between calls and puts.

European vs. American options 

Put-call parity strictly holds for European options, which can only be exercised at expiration.

For American options (which can be exercised anytime) the relationship is more complex. Holders of call options may exercise early to capture dividends, which isn’t factored into the standard formula. Early exercise rights might also impact pricing, as puts and calls are valued differently when exercise is possible before expiration.

IncomeShares ETPs use American-style options. This means early exercise is possible, so put-call parity may not always hold exactly.

Key takeaways 

• Put-call parity defines the relationship between call and put prices with the same strike and expiration.

• The Black-Scholes model builds on this principle, ensuring fair pricing of European options.

• For American options, early exercise can create deviations from the standard